Talk:Pythagorean comma
| This article is rated C-class on Wikipedia's content assessment scale. It is of interest to the following WikiProjects: | ||||||||||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||||||||
Image
[edit]I'm not sure how the image illustrates the Pythagorean comma. Does the image use a scale of cents? Hyacinth (talk) 22:42, 26 July 2008 (UTC)
- ISTM the Pythagorean comma could be much more simply illustrated with a one-dimensional image. The various symbols in the table add nothing and obscure the essential information.
- Hexadecimal, could you do something simpler that only shows pairs of intervals (one just and one ET)?
- For concreteness, it could be annotated with notes of a single scale and specific pitches.
- --Jtir (talk) 11:20, 27 July 2008 (UTC)
Image:MUSICAL SCALES WITH PYTHAGOREAN COMMA.svg listed for deletion
[edit]An image or media file that you uploaded or altered, Image:MUSICAL SCALES WITH PYTHAGOREAN COMMA.svg, has been listed at Wikipedia:Images and media for deletion. Please see the discussion to see why this is (you may have to search for the title of the image to find its entry), if you are interested in it not being deleted. Thank you. Hyacinth (talk) 00:59, 28 July 2008 (UTC) Hyacinth (talk) 00:59, 28 July 2008 (UTC)
Requested audio
[edit]I have added audio examples to the article. Hyacinth (talk) 22:41, 13 August 2008 (UTC) Are the higher and lower versions of the note being played simultaneously, or alternately? I can't hear a difference, and some musician friends say they can't either, so this MID file may be sounding both at once. Could someone supply an audio rendition of the comma which would play the two notes alternately, so we can listen for the pitch difference, then simultaneously for a long enough duration that we can hear the beats (roughly 2 per second at Middle C). Perhaps the existing file does this already, and my ear just isn't good enough to hear it. But in any case the caption should explain what it is doing.CharlesHBennett (talk) 18:49, 11 January 2016 (UTC)
Sign of the Pythagorean comma
[edit]NOTE: This is a spin off of a similar discussion started in another talk page, about the sign of a quantity denoted as ε, which in Pythagorean tuning is supposed to be exactly one twelfth of a Pythagorean comma. In short, we realized that the only way to change the sign of ε was to change the sign of the Pythagorean comma as well, and this is the reason why the discussion was moved here by Glenn L. Paolo.dL (talk) 13:13, 12 August 2010 (UTC)
"Are you proposing to change the definition in Pythagorean comma?"
[edit]The above question was posed to me a few hours ago by frequent music editor Paolo.dL. And yes, I do propose a change in its definition. No, not in its absolute size, but as its currently used reciprocal. Why?
In most meantone temperaments, where the perfect fifth is narrowed by 1/11 of a syntonic comma or more, the sequence of fifths
- G♭-D♭-A♭-E♭-B♭-F-C-G-D-A-E-B-F♯-C♯-G♯
results in the final note G♯, being flatter than its enharmonic counterpart A♭, and F♯ being flatter than its enharmonic counterpart G♭. Most musicians trained to the subtleties of just intonation and meantone temperament understand that this is a natural arrangement and even expect this. The interval between them is a diminished second, the same interval defined as the distance between C and D![]() or between D and E
or between D and E![]() .
.
- In third-comma meantone, F♯ (ratio 25:18) is flatter than G♭ (36:25) by a greater diesis of ratio 648:625.
- In both just intonation and quarter-comma meantone, G♯ (ratio 25:16) is flatter than A♭ (8:5) by a lesser diesis of ratio 128:125.
- In sixth-comma meantone, F♯ (ratio 45:32) is flatter than G♭ (64:45) by a diaschisma of ratio 2048:2025.
- In eleventh-comma meantone, the fifth is flattened to a ratio of approximately 2655:1772 or ≈ 699.9999 cents.
- The resulting F♯ is flatter than G♭ by the approximate ratio of 2479483:2479481 or ≈ 0.0014 cent.
- In 12-tone equal temperament, the fifth is flattened to 700 cents (ratio ≈ 10178:6793). The diminished second of F♯ to G♭ is closed; so are (as one would expect in the equal temperament world) those of B♯ to C to D
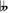 and C
and C to D to E
to D to E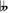 .
.
The problem develops on the other side of the 12-TET line:
- In twelfth-comma meantone, F♯ is now sharper than G♭ by a schisma of ratio 32805:32768.
- And in Pythagorean tuning, the 3:2 perfect fifth is universal.
- The resulting F♯ (ratio 729:512) is also sharper than G♭ (1024:729) by a Pythagorean comma of ratio 531441:524288.
In these last two cases, we have a negative diminished second, and the above "natural arrangement" has been reversed! Ancient Greek ears may have not had a problem with this, but many "just" and "meantone"-trained ears would be surprised, possibly even a bit upset.
In my humble opinion, the best way to illustrate the changeover in the diminished second is to used a "signed" system to define them. If we remove the virtually-equivalent eleventh-comma and 12-TET systems from the remainder of this discussion, five systems remain, each differing from its adjacent system by one-twelfth of a syntonic comma. The corresponding enharmonic notes likewise set a pattern, differing by a full syntonic comma (81:80 or ≈21.50629 cents). Starting with third-comma meantone, one can take the greater diesis as the starting point and subtract successive commas as follows:
- 1/3-c : ≈ +62.56515 cents = 648:625 = greater diesis;
- 1/4-c : ≈ +41.05886 cents = 128:125 = lesser diesis;
- 1/6-c : ≈ +19.55257 cents = 2048:2025 = diaschisma;
- 1/12-c: ≈ - 1.95372 cents = 32768:32805 = schisma;
- Pythag: ≈ -23.46001 cents = 524288:531441 = Pythagorean comma.
The above redefinition for the Pythagorean comma (as well as the schisma) acknowledges that, in the last two cases, the expected diminished-second pairing of G♯ < A♭ fails and is thus a negative interval. A similar case appears at the top of the Pythagorean interval table.
There is an alternative: One can keep the Pythagorean comma and schisma as positive and make the diaschisma and dieses negative instead. However, this might be construed as even more radical than the other way around.
I now invite comments. − Glenn L (talk) 18:11, 14 August 2010 (UTC)
- I will make three points. The worst (and longer) one comes first. Please be patient and read them all with attention when you have time.
- POINT 1.
- What you wrote makes a lot of sense. It reminds me my very first contribution in Wikipedia. It was about the title of an article which I wanted to be changed (Exterior algebra) because it did not make sense (it was a senseless translation from German; it should have been "Extended algebra"!). In a way, I was absolutely right (as you are now), but of course, unfortunately mathematicians accepted that bad translation for centuries, and it is now almost unthinkable to change it, even in the literature (where new ideas are welcome)! So, although your rationale is correct, we cannot change the definition in this article, nor in the articles about the other commas. It would be a violation of two cornerstone policies: Wikipedia:No original research, and Wikipedia:Neutral point of view. These are non-negotiable policies, meaning that even if everyone will agree with you on this page, you cannot do it. And even if you find one book that defines Pythagorean comma or other commas as negative, it would not suffice. From Wikipedia:No original research: "The inclusion of a view that is held only by a tiny minority may constitute original research".
- The Pythagorean comma was known as a positive number since when Euclid computed it, more than two millennia ago, and the other commas are defined in all textbooks as positive. Notice that this does not mean they really are meant to have a direction... In physics, the length (aka magnitude) of a vector, such as the displacement from a point to another, is always positive, even if this vector points in the opposite direction of a Cartesian axis. Indeed, we say that a vector has a length and a direction. Sorry, but I need 2 examples. Please read them: they will prove to be useful.
- Downward is typically a negative direction (because one of the Cartesian axes typically points upward), but the force of gravity has a positive length, and this is why your weight is correctly expressed as a positive number.
- In Newton's third law, which describes the interaction between two objects, action (F) and reaction (R) have the same magnitude, even if they have opposite direction... If you represent these two forces as vectors (each with three scalar components, [Fx, Fy, Fz] or [Rx, Ry, Rz], along three Cartesian axes x, y, z), then
- F = −R
- If you only consider their length or magnitude (a single number), then
- |F| = |R|.
- Notice that there's no such a thing as a negative length. In music, as far as intervals are concerned, you only deal with one dimension (frequency). The frequency domain can be thought as a Cartesian axis, and a musical interval as a displacement vector along that axis. In this case, the vector describing an interval (e.g. from B# to C) would have only one component (along the frequency axis), either negative or positive. Since we are in the frequency domain, the sign is negative when frequency decreases:
- B#-C = −(C-B#)
- However, apparently, music theorists decided to avoid negative numbers! Since frequency ratios by definition give information about the direction of the interval, not only about its length (so, they are equivalent to unidimensional vectors) they decided to swap the order of the operands, so that the size was always positive:
- Pythagorean comma: C-B# (positive, but descending in staff position!)
- Diesis, etc.: B#-C (positive)
- Maybe contemporary theorists are not totally responsible for this decision, as they just were (or felt) forced to accept a previous tradition, but this is what they keep writing in textbooks all over the world, and even on Wikipedia. Your personal opinion, which coincides with mine, is that their choice was not very wise. However, in Wikipedia, expressing a personal point of view in an article is forbidden (see Wikipedia:NPOV), even if many editors agree about that point of view. In short, NOBODY will ever accept such a revolution (unfortunately), neither in the academic world (where original contributions are highly valued), nor in the much more limited world of Wikipedia, where original contributions are forbidden.
- POINT 2.
- Notice that the definition of diminished second on Wikipedia is perfectly compatible with our point of view. Indeed, for instance in Pythagorean interval you can see that the diminished second has a negative sign, and it is exactly the opposite of a Pythagorean comma:
Name Short Ratio Cents ET
Centsdiminished second d2 524288/531441 -23.460 0 Pythagorean comma 531441/524288 23.460 0
- POINT 3.
- Notice also that, whatever is the sign of a comma, when you say "ascend by" or "rise by" or "descend by" or "diminish by" or "augment by", or "sharpen by" or "flatten by" a comma, you specify its direction, which is the same as totally disregarding its sign, and treating it as a length or magnitude, rather than a vector. Perhaps, that's the reason why people does not care about the sign of the Pythagorean comma. The problem arises only when we say: "A diesis is 3 syntonic commas (SC) plus a Pythagorean comma (PC)". It would be nice to say it, but we cannot use algebra in this case, as "plus", "minus", "add" and "subtract", do not imply an increase or decrease in frequency, and do not totally override the sign of the operands. So, as you know, we are forced to say the opposite:
- "A diesis is 3 SC minus a PC"
- or use a sign-overriding terminology, such as
- "A diesis is obtained by moving down 1 PC and up 3 SC".
- The latter would always work, watever is the sign of SC and PC. Thank you for reading it all. Sorry for not being able to make it shorter.
Conclusion
[edit]Thanks for your lengthy response, Paolo. Indeed, your POINT 2 (concerning the Pythagorean diminished second) is precisely why I have taken this stand, as forbidden is it might be outside this Talk page. Be that as it may, I shall take no further action at this point. However, your recent entry here has caused me to place a footnote in this table to note that the Pythagorean diminished second is actually the reciprocal of the Pythagorean comma. − Glenn L (talk) 05:12, 16 August 2010 (UTC)
- This is a typical case, where human language and non-scientific descriptions are rather flexible in using definitions. Mathematically, the various commas and intervals have different signs, but in common usage only positive numbers are used and the direction is circumlocuted. I think the best way to deal with it is to keep the commas and intervals defined as they are. The ε, as being less generally used, may than be signed to solve the issues. However, we must recognise that the diminished second is negative, and in general, that in Pyhagorean, flats are lower than sharps (we get ascending G-Ab-G#-A). −Woodstone (talk) 08:56, 16 August 2010 (UTC)
- You taught me a new world (circumlocuted)! Thank you for your comment. So, I guess I can say that we all agree now about what follows:
- The Pythagorean d2 is negative
- The Pythagorean comma is positive
- This image, which I inserted in the introduction, summarizes our conclusion.
Pythagorean comma (PC) defined in Pythagorean tuning as difference between semitones (A1-m2), or interval between enharmonically equivalent notes (from D♭ to C♯). The diminished second has the same width but an opposite direction (from to C♯ to D♭). - − Paolo.dL (talk) 16:04, 16 August 2010 (UTC)
- You taught me a new world (circumlocuted)! Thank you for your comment. So, I guess I can say that we all agree now about what follows:
Sign of ε
[edit]I copied here a comment by Glenn L, which in my opinion is extremely effective (especially in what he calls a "poorly-edited number line"), and was posted in Talk:Wolf interval before we realized that the sign of ε was connected with the sign of the Pythagorean comma, and decided to move the discussion here (see above). Paolo.dL (talk) 15:14, 17 August 2010 (UTC)
- The problem is that in most meantone temperaments (up to eleventh-comma), the ε is subtracted from the 700-cent fifth, whereas in twelfth-comma and Pythagorean tuning it is added to the fifth. That is precisely why I defined the greater/lesser diesis, diaschisma (all above unison), schisma & Pythagorean comma (both below unison) the way I did. The ε, in effect, changes sign when crossing the 700-cent line a micrometer (!) from the eleventh-comma mark. Let's see if this poorly-edited number line (the letter "e" for equal temperament should be almost precisely in the middle of the digits "11" for 11th-comma, otherwise nearly to scale) can help illustrate the problem:
- Pythag||||||||||||||||||||||||||||||12|e11|||||||||||||||||||||||||6th|||||||||||||||||||||||||||||||||4th|||||||||||||||||||||||||||||||||3rd
- On the number line, Pythagorean ≈ 701.95500 cents; 12[th-comma] ≈ 700.16281; "e[t]" = 700.00000; 11[th-comma] ≈ 699.99988; 6th[-comma] ≈ 698.37062; 4th[-comma] ≈ 696.57843; and 3rd[-comma] ≈ 694.78624. I'm using 5 decimals only because ET and 11th-comma are so close together; otherwise, 1 decimal is sufficient (702.0, 700.2, 700.0 twice, 698.4, 696.6 and 694.8 cents respectively).
- The distances between Pythagorean & 12th-c, 12th-c & 6th-c, 6th-c & 4th-c, and 4th-c & 3rd-c are precisely 1/12-comma ≈ 1.79219 ≈ 1.8 cents by definition. For all systems to the right of the "e" mark, the corresponding fifth is narrower than 700 cents, so ε must be subtracted from 700. for those systems to the left of "e", the corresponding fifth is wider than 700 cents, so ε must be added to 700. Thus we need to present ε in a different way on either side of the line.
- — Glenn L (talk) 03:14, 9 August 2010 (UTC)
In Talk:Wolf interval we agreed with Glenn L that the sign of ε should be, for the sake of consistency, the same as the sign of the comma. And indeed we defined it everywhere as 1/12 of the comma. Moreover, that's the very reason why we moved here, from there, the discussion about the sign of ε, and turned it into a discussion about the sign of the Pythagorean comma (PC). So, can we all agree now about what follows?
- ε is defined as 1/12 of a comma, and hence is also always positive.
− Paolo.dL (talk) 16:04, 16 August 2010 (UTC)
- I agree that:
- The Pythagorean d2 is negative, and
- The Pythagorean comma is positive. However, since the sign of d2 is what is important, I'd like to propose that
- ε be defined as 1/12 of the diminished second in all regular tuning systems.
- Its sign, like that of the corresponding d2, would be:
- Negative from Pythagorean tuning, past 53-tone equal temperament and twelfth-comma meantone, to just short of 12-tone equal temperament;
- Zero for 12-tone equal temperament (since d2 is tonal unison, and therefore it and ε = 0 cents); and
- Positive from eleventh-comma meantone (where d2 ≈ +1/716.1 cent) onwards, including quarter-comma meantone.
- − Glenn L (talk) 00:35, 17 August 2010 (UTC)
POINT 1.
I have a few questions for you: what is the reason why you moved the discussion about the sign of ε here, and turned it into a discussion about the sign of the Pythagorean comma (PC)? What's the connection between ε and PC, if you don't want to define ε as 1/12 of a comma? And more importantly, what was the reason why you chose to discuss PC before ε? These questions may seem not useful in this context, because you may simply answer that you changed your mind, and you are perfectly entitled to do so, or that you just wanted commas to be consistent with ε (rather than vice versa). But please indulge me, and think about the reason why you wanted to discuss PC before ε.
ε, in my opinion, does not need to be a unidimensional vector. It works better as a length (see my comment above). In other words, it does not need to have a direction, as a negative sign would only confuse the reader in the context where ε is used. ε is simply used to define intervals within a single tuning system (e.g., right at the end of this section). So, it is convenient to define it as 1/12 of a comma, i.e. as a length, with positive sign. You may ask: what's the difference between a comma and an ε, then? Your answer to my question above might give you insight about this: the difference is that ε is not used for comparisons between tuning systems. For that, we use commas (see table in comma (music)), or fifths. Even your "poorly edited number line", that I copied above, is actually a comparison between the sizes of fifths, not between the sizes of ε, and that's why I like it!
As I wrote in the previous section, I would prefer the PC to be negative, which would imply a negative ε. But this does not mean that I like a negative ε! It would not be wise. The reader would not understand the negative sign in the context where ε is used. Namely, ε is defined at the end of this section as the difference between the size of the fifth and 700:
| Definition of ε in Pythagorean tuning |
|---|
| By definition, in Pythagorean tuning 11 perfect fifths have a size of approximately 701.955 cents (700+ε cents, where ε ≈ 1.955 cents). |
This definition is already crystal clear, and in my opinion it is highly desirable to leave it as it is, as simple as possible. The fact that 701.955 is larger than 700 is so clear, in this definition, that I really can't see the need to give ε a negative sign. It would only decrease readability, with no advantage whatsoever. This is also true in other contexts, such as here, here, or here.
In short, the only advantage of having PC positive, is to have ε positive as well!
− Paolo.dL (talk) 11:27, 17 August 2010 (UTC)
POINT 2.
By the way, the direction of ε is absolutely clear in this definition, and it happens to be positive (increasing frequency)! So, it does not make sense to give ε a negative sign. However, I guess it might make some sense (but it would slightly decrease readability) to define ε as negative in meantone temperaments where the fifth is tempered to a size narrower than 700 cents. But we cannot do it, because we agreed that, according to the literature, in those tuning systems both d2 and the comma are defined as positive!
I am not implying, however, that this discussion was useless. It is useful to think and be aware about this problem, and this discussion, together with the previous about the sign of PC, already produced a few good edits, even though we are limited by Wikipedia policies.
− Paolo.dL (talk) 14:54, 17 August 2010 (UTC)
- I agree that it may be more readable to keep ε positive in all cases. Unfortunately, it is also inconsistent, because what is, for example, 700−ε in meantone temperaments such as 1/4-comma meantone becomes 700+ε in Pythagorean tuning. And while I agree (because of universal use in music literature) that the Pythagorean comma and schisma, like all other commas, are positive intervals (see my recent edits to your comma table), I still draw the line on the sign of ε. True, while ε is generally positive in mathematical literature, it is seldom if ever encountered in music literature. I guess the question is: Which is better, consistency or readability? I know we don't want to confuse anyone, but it appears that some may be confused no matter what is decided. Since commas vary tremendously in definition, but the diminished second does not, I prefer defining ε in terms of the diminished second − even when the latter is negative as in Pythagorean tuning. − Glenn L (talk) 16:33, 17 August 2010 (UTC)
I like your edits on the table in Comma (music), as I like all your (and my) edits inspired by this discussion, but when you use this example here you seem to forget (again, as in your "poorly-edited number line") that commas or fifths are used to compare tuning systems, while ε was created for a totally different reason.
You maintain that to keep ε always positive is "inconsistent". Inconsistent with what? Yes, it is inconsistent with d2, but perfectly consistent with commas. And, consistent with itself as well, its consistence residing in its lack of direction (i.e. in its definition as a length, which is perfectly compatible with mathematics)... Anyway, I disagree that it should be consistent with d2 or with commas. Contrary to what I wrote at the beginning of this discussion, I am now convinced that the main definition of ε is not and should not be based on commas or d2. The correct definition in Pythagorean tuning is given above. One way to summarize my main point is this: ε does not need to be consistent between tuning systems, but only within each tuning system. (BTW, otherwise it would be wise to give it a different symbol for each tuning system.)
However, let's imagine for a moment that ε needed to be also consistent between tuning systems, as you suggest. In this case, as I showed above, in my opinion a reasonable definition of ε would be:
In other words, ε was created to represent the amount by which P5 deviates from 700 (which is the average size for the 12 fifths in 12-tone scales) within a given tuning system. And that definition currently is and should remain the main one, the first one, in all contexts where ε is defined, because it proves to be useful to make the concept crystal clear to the reader. We created ε only to show that, within the tuning system in which it is defined, any interval deviates from the relevant average by a multiple of ε. This is its sense of life, written in its "Genesis" book. We did not create it to compare tuning systems. And this implies that:
This is what logic would dictate if and only if we needed to be also consistent between tuning systems, and it is the opposite of what you propose:
However,
- typically, musicians are not interested in abstract logic (this is an understatement :-), and
- even this approach would decrease readability, and would confuse the reader, who does not care a damn about the comparison between tuning systems when trying to understand that all intervals, in a specific tuning system, deviate from average by a multiple of a constant quantity. This is what we want them to understand when we use ε. When we use ε, we should focus on that purpose. The information that other tuning systems have P5 on the other side of 700 is secondary, it is not pertinent, it is not given in the same section, it is given only in articles in which tuning systems are compared!
So, because of this, and also because of what I wrote in my POINT 2 above, it might be reasonable to give ε a negative sign in tuning systems with P5 < 700 cents (which is the opposite of what you suggest), but in my opinion we can't do it, because it is more reasonable to keep it always positive, as it always was and still is on Wikipedia. The opposite, i.e. assigning to ε the same sign as d2, is even less reasonable, although mathematically it would work all the same. And please do not forget that the current definition is mathematically correct as well!
− Paolo.dL (talk) 19:06, 17 August 2010 (UTC)
- You have correctly stated: "ε does not need to be consistent between tuning systems, but only within each tuning system. (BTW, otherwise it would be wise to give it a different symbol for each tuning system.)" Okay, let's keep ε for most meantone systems where P5 < 700 cents, so that ε = 700 - P5 is always positive. Then we can use another Greek letter, δ, for systems like 1/12-comma and Pythagorean where P5 > 700 cents, so that δ = P5 - 700 is also always positive. I can go for that. Or we can go the other way: ε when P5 > 700 and δ when P5 < 700. − Glenn L Glenn L (talk) 20:20, 17 August 2010 (UTC)
- I am sure you agree that the paragraphs where ε is used are already crystal clear, they do not create doubts. Your need for abstract consistency "between" tuning systems comes from your deep knowledge of the relations between tuning systems (something that I am glad you shared with me), but there's nothing ambiguos in the text we are discussing, and even people with your knowledge, believe me, will never feel the need to think about complex relations between tuning systems when studying separately the distribution of intervals in each single tuning system, as in this case. Even the "direction of ε" (provided you want to see it as a vector, rather than a length) is already crystal clear: have you noticed that there's always a minus before ε wherever P5 < 700, and a plus wherever P5 > 700? A sign "outside" ε is even clearer than "inside" it.
- However, I am neutral about your suggestion of using δ for Pythagorean tuning. This means that, although I cannot see the need for correcting a text that in my opinion is already perfect, I have nothing against it. And you don't need to convince me. There are much more important problems to solve. Articles or sections that are so badly written that they create new doubts, or mislead the readers, rather than helping them to understand. For instance, have you seen how messy are the articles about meantone temperament and syntonic temperament? I think they need to be rewritten almost from scratch (but I have not enough time or knowledge to do it). They mix up even me.
- A question for you. Do you have any particular reason for using δ (
δ) or ε (ε), rather than δ and ε, provided as special characters in Wikipedia standard editor? If not, I suggest you to use the special characters, because they make edits easier. Paolo.dL (talk) 18:08, 18 August 2010 (UTC)
This article is the absolute worst
[edit]Totally useless for anyone not already a complete expert in music theory. First section needs an entire rewrite. —Preceding unsigned comment added by 195.112.40.182 (talk) 09:48, 28 November 2010 (UTC)
- To understand this material, it is necessary to spend some time reading separate articles. Otherwise, we fall in to the well-known wikipedia difficulty of trying to say everything in one article. I believe it to be the consensus of Wikipedia users that it's better to have well-factored, multiple, shorter articles and to flip between them in your browser tabs rather than being compelled to scroll through everything in one massive article. The entry price to this article is, after all, understanding that tunings are mathematical systems, and if you understand that and have enough math to make thinking about Pythagorean tuning a profitable pastime, the article is actually pretty easy to follow. JacquesDelaguerre (talk) 07:18, 3 December 2010 (UTC)
- That's a good way to put it, Jaxdelaguerre. Isn't this the point of hyperlinks are, after all? I understand this page quite well, but then I already understand the pages linked to pretty well. In contrast, I can't make heads or tails of Hessian matrix. But it would be awkward, and unnecessary, for that page to start out explaining matrix algebra (or whatever it is about). Now I'm wondering, is there a Wikipedia guideline or essay on this topic? Pfly (talk) 07:38, 3 December 2010 (UTC)
- Math pages can indeed be pretty obscure, however, hyperlinks (as you noted above) allow the reader to wander around the topic space and to self-educate. The Wikipedia page "Help:Wikipedia: The Missing Manual/Building a Stronger Encyclopedia/Better Articles: A Systematic Approach" lightly covers in an introductory fashion a lot of Wikipedia design patterns. Perhaps good factoring and a bias towards clarity and simplicity are the most important design patterns for Wikipedia authors. - JacquesDelaguerre (talk) 16:00, 3 December 2010 (UTC)
- fwiw the intro has improved since my first comment, the first para is much more approachable and the reference in the second to fifths and octaves is helpful.195.112.40.182 (talk) 10:55, 11 December 2010 (UTC)
- Math pages can indeed be pretty obscure, however, hyperlinks (as you noted above) allow the reader to wander around the topic space and to self-educate. The Wikipedia page "Help:Wikipedia: The Missing Manual/Building a Stronger Encyclopedia/Better Articles: A Systematic Approach" lightly covers in an introductory fashion a lot of Wikipedia design patterns. Perhaps good factoring and a bias towards clarity and simplicity are the most important design patterns for Wikipedia authors. - JacquesDelaguerre (talk) 16:00, 3 December 2010 (UTC)
- That's a good way to put it, Jaxdelaguerre. Isn't this the point of hyperlinks are, after all? I understand this page quite well, but then I already understand the pages linked to pretty well. In contrast, I can't make heads or tails of Hessian matrix. But it would be awkward, and unnecessary, for that page to start out explaining matrix algebra (or whatever it is about). Now I'm wondering, is there a Wikipedia guideline or essay on this topic? Pfly (talk) 07:38, 3 December 2010 (UTC)
Any actual suggestions instead of empty criticisms? Hyacinth (talk) 11:52, 11 December 2010 (UTC)
Images showing octave + comma
[edit]In some articles about specific commas (this article, Comma (music), and Syntonic comma ), and in the article about semitone there are pictures showing, in staff notation, an octave + a comma (or semitone), rather than simply a comma (or semitone). On the other hand, the attached audio file typically plays a true comma (not a comma + octave). I tried to remove the picture recently added in this article. And I carefully explained the reason in my edit summary:
- The notes on staff showed interval = 1 octave + 1 PC (pitch class not clear in staff notation). I removed picture (hoping that you can draw and insert a correct one), but I kept the audio file.
However, my edit was unpolitely reverted without explanation.
— Paolo.dL (talk) 17:50, 3 December 2010 (UTC)
- I have edited to make it say what you and I believe to be the truth. Maybe someone will make a new clip or a new image so they match. JacquesDelaguerre (talk) 03:44, 4 December 2010 (UTC)
- That's better, thank you. But I believe a picture like that is at least useless. What's the reason why an article about a comma should show an image of an octave plus that comma? How does that help the reader to understand the comma? I believe it does not. Moreover, it forces the reader to waste its time to read the long caption just to understand that the picture does not show a comma. The reader will eventually understand that the picture is useless. Again, isn't this a waste of time?
- This is against a principle that you expressed in the previous section, which should be applied, in my opinion, in all the above mentioned articles: "Perhaps good factoring and a bias towards clarity and simplicity are the most important design patterns for Wikipedia authors".
- —Paolo.dL (talk) 08:44, 4 December 2010 (UTC)
- Paolo, I agree with you completely, it is not I who is reverting your changes. I hope you or someone else with digital talent will change the image and/or the sound file and make them consistent with one other! JacquesDelaguerre (talk) 17:50, 4 December 2010 (UTC)
I do not have a software to produce the image. And my point is not exactly the same as yours. You seem to wish only consistency, but an audio file consistent with that picture would be as useless as the picture! I do not need only consistency, but mainly "semplicity and clarity", and pictures and audio files which make the concept clear rather than creating doubts. So, I suggest to delete the picture, and just use the audio file, in all the above mentioned articles. I am not going to do that alone, as I hate edit wars with unpolite people.
I was hoping that Hyacinth, the author of the pictures, would understand my point and fix them, but unfortunately he is also the editor that reverted my edit without explanation, and even ignored my polite request in his talk page.
So, I thank you for your kind support, but I am asking you and other editors to answer this question, regarding all the above-mentioned articles: while we wait for somebody (hopefully the original author) to draw new pictures showing the correct intervals (it may take long, as the author is ignoring my request), is it better to keep those pictures or delete them? — Paolo.dL (talk) 19:24, 4 December 2010 (UTC)
- For what it's worth, it wouldn't be that hard to create music notation images like these with GNU LilyPond. Pfly (talk) 05:28, 5 December 2010 (UTC)
- Hyacinth, thank you for fixing the pictures:
In this article In Comma (music) and
Syntonic commaIn Semitone#Just intonation 
Pythagorean comma on C . 
Syntonic comma on C . 
'Larger' or major limma on C .
Audio sample correct?
[edit]Don't mean to quibble, but is the sample correct? I only hear a unison. 23.6 cents should make an audible difference, no? The syntonic comma sample is distinct at a slightly narrower interval. — Preceding unsigned comment added by 68.47.101.16 (talk) 01:02, 20 August 2015 (UTC)
- Should be correct now. Hyacinth (talk) 23:39, 11 January 2016 (UTC) Thanks for pointing out the error. Hyacinth (talk) 08:00, 12 January 2016 (UTC)
- @Hyacinth: Thanks, the new midi file plays correctly on my system. Looking at the history of , you also changed the pitch bend event values from "65,71" to "6,71". By my calculations,[1] the previous values were consistent with the article. Can you explain this change? Burninthruthesky (talk) 14:14, 1 February 2017 (UTC)
References
- ^ (65+71*2^7-8192)/4096 = 0.2346
(6+71*2^7-8192)/4096 = 0.2202
- See: Help:Sibelius#MIDI pitch bend.
- 0 cents = 0,64, and each byte has a range from 0-127.
- pitch bend cents = X,Y = (X*(1/(128*32))) + ((Y-64)*(100/32)) = (X*.25) + ((Y-64)*3.125)
- Thus "6,71" = 1.5 + 21.875 = +23.38 cents, 0+23.38 = 23.38 cents, our goal being 23.46.
- Hyacinth (talk) 04:45, 2 February 2017 (UTC)
- Thanks for the explanation. Misplacing a decimal point is easily done. 1/(128*32) = 0.0002441, which is ≈ 0.025 percent, not 0.25. The corrected version of the above calculation is:
- 65*0.02441+(71-64)*3.125 = 23.46
- Burninthruthesky (talk) 09:26, 2 February 2017 (UTC)
- Thanks for the explanation. Misplacing a decimal point is easily done. 1/(128*32) = 0.0002441, which is ≈ 0.025 percent, not 0.25. The corrected version of the above calculation is:
- Misplacing a decimal point may easily be done, but it was not done in this case. I believe that 0.25 came from 128/32. You may have noticed that 0.25 and .0002441 are more than one decimal point apart. Hyacinth (talk) 00:30, 9 March 2017 (UTC)
- Yes. Sorry if "≈ 0.025 percent" was unclear. Burninthruthesky (talk) 07:26, 11 March 2017 (UTC)
- Misplacing a decimal point may easily be done, but it was not done in this case. I believe that 0.25 came from 128/32. You may have noticed that 0.25 and .0002441 are more than one decimal point apart. Hyacinth (talk) 00:30, 9 March 2017 (UTC)
- Thanks for pointing out the error(s). This may take a while to fix, but, if it is any comfort, the Pythagorean comma, for example, was only (23.46-22.02=) 1.44 cents off. Hyacinth (talk) 05:22, 11 March 2017 (UTC)
- Some of these errors are small, so are the differences we are trying to demonstrate. Thanks for your continuing help with the clean up. Burninthruthesky (talk) 07:26, 11 March 2017 (UTC)
- Yes, 1.44 cents may be less than the just noticeable difference, but is about a sixteenth of the Pythagorean comma. I would say that it is to your credit that you noticed. Hyacinth (talk) 22:23, 11 March 2017 (UTC)
- I noticed because some time ago (before the Jan 2016 fix) I believed I couldn't hear the interval of a Pythagorean comma. It was only recently, when experimenting with MIDI, I realized I'd been misinformed by this article. Burninthruthesky (talk) 09:32, 13 March 2017 (UTC)
- You knew even though the article lied to you. It's too bad you didn't write the article. Hyacinth (talk) 00:14, 27 March 2017 (UTC)
- I noticed because some time ago (before the Jan 2016 fix) I believed I couldn't hear the interval of a Pythagorean comma. It was only recently, when experimenting with MIDI, I realized I'd been misinformed by this article. Burninthruthesky (talk) 09:32, 13 March 2017 (UTC)
- Yes, 1.44 cents may be less than the just noticeable difference, but is about a sixteenth of the Pythagorean comma. I would say that it is to your credit that you noticed. Hyacinth (talk) 22:23, 11 March 2017 (UTC)
- Some of these errors are small, so are the differences we are trying to demonstrate. Thanks for your continuing help with the clean up. Burninthruthesky (talk) 07:26, 11 March 2017 (UTC)
- Thanks for pointing out the error(s). This may take a while to fix, but, if it is any comfort, the Pythagorean comma, for example, was only (23.46-22.02=) 1.44 cents off. Hyacinth (talk) 05:22, 11 March 2017 (UTC)
MIDI errors (2)
[edit]Following the above discussion, we have the MIDI file as accurate as possible using the current method. As stated here, a Pythagorean comma = 23.460010 cents. At 23.461914 cents, the interval represented in the MIDI file is not accurate to six decimal places. This is an example of false precision. I have again corrected this error. Burninthruthesky (talk) 07:39, 28 March 2017 (UTC)
In view of the ongoing edits, I have posted a request for help at the Mathematics WikiProject. Burninthruthesky (talk) 07:58, 28 March 2017 (UTC)
Re "the file is an estimation of a Pythagorean comma". It doesn't say it's an "estimation", and in fact it isn't. Even if it were properly explained, I still don't believe a discussion of the limits of MIDI precision belongs in this article. As pointed out in the discussion above, it is below the just noticeable difference. Is there any WP:CONSENSUS for this edit? Burninthruthesky (talk) 08:56, 28 March 2017 (UTC)
- Not sure I understand the matter. But for the best of my understanding, we take the ratio r = 312/219 and express it in cents, which means If so, then my calculation gives 23.4600103... cents. Boris Tsirelson (talk) 09:00, 28 March 2017 (UTC)
- Thanks, I agree with your calculation, and I don't think the correct value is really in dispute. The dispute centres around the linked MIDI file which, for various technical reasons, has previously not played the correct interval. Now it is as correct as possible (in fact it is "23.461914 cents") there is a dispute over whether the article should describe the file to that level of precision. Burninthruthesky (talk) 09:11, 28 March 2017 (UTC)
- Ah... Then, this is not a question to a mathematician. If you want the reader to know only that the file is nearly correct, then the answer is "no". If you also want the reader to know the small incorrectness of this file (beyond acoustic recognition), then the answer is "yes, but 23.462 would be enough". Boris Tsirelson (talk) 09:28, 28 March 2017 (UTC)
- Yes, that seems reasonable. Currently the article body says "approximately 23.46 cents". This is consistent with both the MIDI file and the mathematically correct value. Burninthruthesky (talk) 09:45, 28 March 2017 (UTC)
- "Approximately 23.46 cents" sounds ok to me. My understanding is that thousandths of a cent are well below the differences that humans can perceive. —David Eppstein (talk) 17:54, 29 March 2017 (UTC)
- Yes, that seems reasonable. Currently the article body says "approximately 23.46 cents". This is consistent with both the MIDI file and the mathematically correct value. Burninthruthesky (talk) 09:45, 28 March 2017 (UTC)
- Ah... Then, this is not a question to a mathematician. If you want the reader to know only that the file is nearly correct, then the answer is "no". If you also want the reader to know the small incorrectness of this file (beyond acoustic recognition), then the answer is "yes, but 23.462 would be enough". Boris Tsirelson (talk) 09:28, 28 March 2017 (UTC)
- Thanks, I agree with your calculation, and I don't think the correct value is really in dispute. The dispute centres around the linked MIDI file which, for various technical reasons, has previously not played the correct interval. Now it is as correct as possible (in fact it is "23.461914 cents") there is a dispute over whether the article should describe the file to that level of precision. Burninthruthesky (talk) 09:11, 28 March 2017 (UTC)
- A difference of about 1.44 cents was just heard. The JND for pitch may be as high as 10 cents. More important may be the precision of MIDI files. Hyacinth (talk) 00:46, 4 April 2017 (UTC) Hyacinth (talk) 00:47, 4 April 2017 (UTC)
- I have reverted this incorrect edit. It is not an "estimate". We know precisely what the interval of a Pythagorean comma should be, and the data in the MIDI file is as accurate as it can be. The inaccuracy in this case is 0.0019 cents. I see no support here for referring to that in the article. Burninthruthesky (talk) 06:57, 4 April 2017 (UTC)
- C-Class Greek articles
- Mid-importance Greek articles
- WikiProject Greece general articles
- All WikiProject Greece pages
- C-Class Classical Greece and Rome articles
- Mid-importance Classical Greece and Rome articles
- All WikiProject Classical Greece and Rome pages
- C-Class mathematics articles
- Mid-priority mathematics articles










